|
Texture
In works Memo |
Maud /
Define The Compression Direction< Lattice preferred orientations | Radial Diffraction | Using pole figures to represent directions in space > Data coverage in axial and radial experimentsExplaining data coverage takes a lot of figures, so I moved it into separate sections:
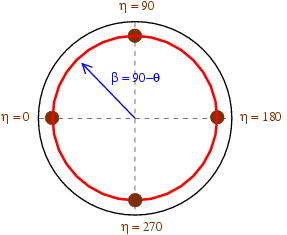
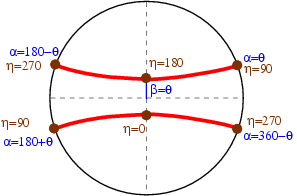
In summary, the data coverage from one diffraction ring should look like the figure below. Direction of compression is in the center, data coverage is in red, blue indicate values for the angle alpha and beta, and brown is used to locate points corresponding to specific azimuth on the imaging plate.
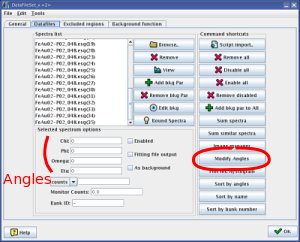
In the axial geometry, all orientations on a diffraction ring are located at the same distance from the compression direction, In the radial geometry, various orientations on the diffraction ring probe different distances of the compression direction. If one assumes an axial symmetry around the compression direction, we probe the all space of orientations. Finding out orientation coverage in MaudWhen running MAUD, they can be issues with the orientations of your datafiles. Things can be rotated within MAUD, and experiments are not always performed in the same geometry. To find out your coverage, the easiest way I know off is to to use the texture functions. Open the
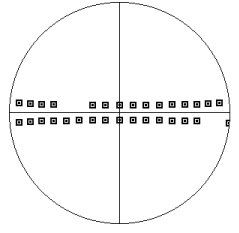
If you're dealing with a radial diffraction experiment and your coverage looks like Figure 1, something is wrong with your orientations. It should look like Figure 4. Locating the compression directionThe next step is to locate your compression direction. It should be at the center of the pole figure, otherwise, things will not work properly:
In order to check that your compression direction is ok,
If the square that disappeared was on the outside rim, something is wrong with your orientations: the data for the compression direction does not point to the center of pole figure. Fixing things out
There are two ways to bring you data into the proper orientation
In the dataset window, The I do not have a good way to do this: just find the angles that will bring you the orientation you want. There are multiple solutions and it depends on your experimental setup... |